Variational series

series of order statistics

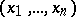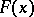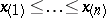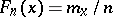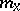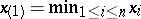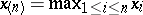An arrangement of the values of a random sample with distribution function in ascending sequence . The series is used to construct the empirical distribution function , where is the number of terms of the series which are smaller than . Important characteristics of series of order statistics are its extremal terms (, ) and the range 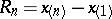. The densities of the distributions of the minimum and maximum terms of a series of order statistics in the case

are defined by the expressions

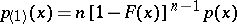and

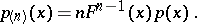Considered as a stochastic process with time index 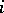, , 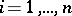the series of order statistics forms a non-homogeneous Markov chain.

References

[1]	S.S. Wilks, "Mathematical statistics" , Wiley (1962)

Comments

The phrase "variational series" is almost never used in the West. Cf. also Order statistic.

References

[a1]	E.L. Lehmann, "Testing statistical hypotheses" , Wiley (1986)