Metric dimension
A numerical characteristic of a compact set, defined in terms of coverings of "standard measure" , the number of which defines the metric dimension. Let  be a compact set, and let
be a compact set, and let  be the minimal number of sets with diameter not exceeding
be the minimal number of sets with diameter not exceeding  that are needed in order to cover
that are needed in order to cover  . This function, depending on the metric in
. This function, depending on the metric in  , takes integer values for all
, takes integer values for all  , and increases without bound as
, and increases without bound as  ; it is called the volume function of
; it is called the volume function of  . The metric order of the compact set
. The metric order of the compact set  is the number
is the number
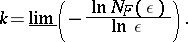 |
This quantity is not yet a topological invariant. Thus, the metric order of a curve in the sense of Jordan (cf. Line (curve)) with the Euclidean metric is equal to 1, but for a curve in the sense of Jordan passing through a perfect totally-disconnected set in  of positive measure, this value is equal to
of positive measure, this value is equal to  . However, the greatest lower bound of the metric orders for all metrics on
. However, the greatest lower bound of the metric orders for all metrics on  (called the metric dimension) is equal to the Lebesgue dimension (the Pontryagin–Shnirel'man theorem, 1931, see [1]).
(called the metric dimension) is equal to the Lebesgue dimension (the Pontryagin–Shnirel'man theorem, 1931, see [1]).
References
| [1] | W. Hurevicz, G. Wallman, "Dimension theory" , Princeton Univ. Press (1948) |
Comments
Metric dimension makes sense for non-compact separable metrizable spaces (using totally bounded metrics), and the Pontryagin–Shnirel'man theorem extends to them. This was shown by E. Szpilrajn-Marczewski. See [a2].
There are also other types of metric-dependent dimension functions.
One example is the Hausdorff dimension.
Another example is obtained by modifying the definition of the covering dimension  (see Dimension): If
(see Dimension): If  is a metric space, one defines
is a metric space, one defines  by
by  if and only if for every
if and only if for every  there is an open covering
there is an open covering 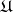 of
of 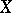 with
with  and
and 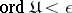 . Here
. Here  and
and  means that no point of
means that no point of  is an element of more than
is an element of more than  elements of
elements of  . One can show that
. One can show that  and that these inequalities are best possible, see [a1].
and that these inequalities are best possible, see [a1].
References
| [a1] | R. Engelking, "Dimension theory" , North-Holland & PWN (1978) pp. 19; 50 |
| [a2] | J.-I. Nagata, "Modern dimension theory" , Interscience (1965) |
Metric dimension. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Metric_dimension&oldid=14147