Uniformly most-powerful test
A statistical test of given significance level for testing a compound hypothesis 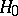 against a compound alternative
against a compound alternative  , whose power is not less than the power of any other statistical test for testing
, whose power is not less than the power of any other statistical test for testing 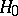 against
against 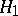 of the same significance level (cf. Power of a statistical test).
of the same significance level (cf. Power of a statistical test).
Suppose that a compound hypothesis 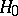 :
: 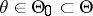 has to be tested against the compound alternative
has to be tested against the compound alternative 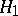 :
: 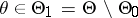 , and there is given an upper bound
, and there is given an upper bound  ,
, 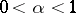 , for the probability of an error of the first kind, made by rejecting
, for the probability of an error of the first kind, made by rejecting 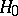 when it is in fact true (the number
when it is in fact true (the number 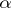 is called the significance level of the test, and it is said that the test has level
is called the significance level of the test, and it is said that the test has level 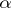 ). In this way, the restriction on the probability of an error of the first kind reduces the set of tests for testing
). In this way, the restriction on the probability of an error of the first kind reduces the set of tests for testing 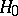 against
against 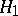 to the class of tests of level
to the class of tests of level  . In terms of the power function (cf. Power function of a test)
. In terms of the power function (cf. Power function of a test) 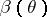 ,
, 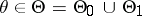 , a statistical test of fixed significance level
, a statistical test of fixed significance level 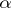 means that
means that
 |
If, in the class of all tests of level 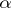 for testing
for testing 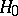 against
against 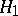 , there is one whose power function
, there is one whose power function  satisfies
satisfies
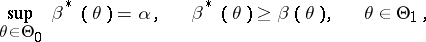 |
where 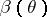 is the power function of any other test from this class, then this test is called a uniformly most-powerful test of level
is the power function of any other test from this class, then this test is called a uniformly most-powerful test of level 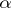 for testing
for testing 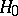 against
against  . A uniformly most-powerful test is optimal if the comparison is made in terms of the power of tests.
. A uniformly most-powerful test is optimal if the comparison is made in terms of the power of tests.
References
| [1] | E.L. Lehmann, "Testing statistical hypotheses" , Wiley (1959) |
Uniformly most-powerful test. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Uniformly_most-powerful_test&oldid=13669