Covering

A mapping of a space onto a space such that each point has a neighbourhood the pre-image of which under is a union of open subsets that are mapped homeomorphically onto by . Equivalently: is a locally trivial fibre bundle with discrete fibre.

Coverings are usually considered on the assumption that 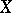and 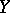are connected; it is also usually assumed that is locally connected and locally simply-connected. Under these assumptions one can establish a relationship between the fundamental groups 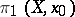and : If , then the induced homomorphism 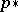maps 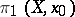isomorphically onto a subgroup of 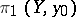and, by varying the point 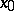in 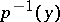, one obtains exactly all subgroups in the corresponding class of conjugate subgroups. If this class consists of a single subgroup 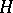(i.e. if is a normal divisor), the covering is said to be regular. In that case one obtains a free action of the group 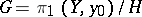on 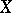, with 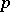playing the role of the quotient mapping onto the orbit space 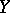. This action is generated by lifting loops: If one associates with a loop 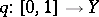, , 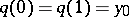the unique path 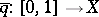such that 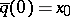and 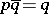, then the point 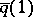will depend only on the class of the loop in 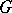and on 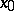. Thus, each element of corresponds to a permutation of points in . This permutation has no fixed points if 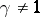, and it depends continuously on . One obtains a homeomorphism of .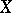

In the general case this construction defines only a permutation in 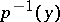, i.e. there is an action of on 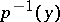, known as the monodromy of the covering. A special case of a regular covering is a universal covering, for which 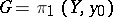. In general, given any subgroup , one can construct a unique covering 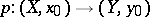for which 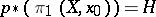. The points of 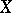are the classes of paths 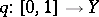, 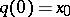: Two paths 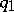and 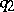are identified if 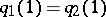and if the loop lies in an element of . The point 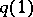for the paths of one class is taken as the image of this class; this defines . 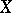The topology in is uniquely determined by the condition that 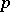be a covering; it is here that the local simple-connectedness of 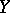is essential. For any mapping 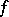of an arcwise-connected space 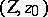into 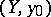, its lifting into a mapping 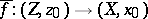exists if and only if 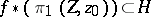. A partial order relation can be defined on the coverings of (a covering of a covering is a covering); this relation is dual to the inclusion of subgroups in 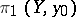. In particular, the universal covering is the unique maximal element.

Examples. The parametrization 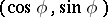of the circle defines a covering of the circle by the real line, 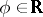, often described in the complex form 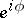and called the exponential covering. Similarly, the torus is covered by the plane. Identification of antipodal points on a sphere yields a covering by the sphere of a projective space of corresponding dimension. In general, free actions of discrete groups are a source of regular coverings (over the orbit space); not every such action yields a covering (the orbit space may be non-separable), but finite groups do.

Comments

A covering is also a termed a covering projection. Every covering has the homotopy lifting property (cf. Covering homotopy) and hence is a Hurewicz fibre space or fibration.

References

[a1]	E.H. Spanier, "Algebraic topology" , McGraw-Hill (1966) pp. Chapt. 2