Imprimitive group

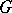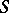A group of one-to-one mappings (permutations, cf. Permutation) of a set onto itself, for which there exists a partition of into a union of disjoint subsets 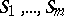, , 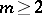with the following properties: the number of elements in at least one of the sets 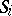is greater than ; for any permutation 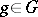and any , , there exists a 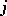, , 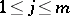such that 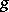maps 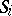onto .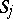

The collection of subsets 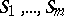is called a system of imprimitivity, while the subsets 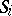themselves are called domains of imprimitivity of the group 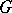. A non-imprimitive group of permutations is called primitive.

An example of an imprimitive group is a non-trivial intransitive group of permutations of a set 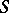(see Transitive group): for a system of imprimitivity one can take the collection of all orbits (domains of transitivity, cf. Orbit) of 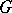on 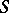. A transitive group of permutations of a set 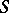is primitive if and only if for some element (and hence for all elements) 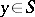the set of permutations of 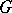leaving 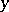fixed is a maximal subgroup of .

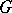The notion of an imprimitive group of permutations has an analogue for groups of linear transformations of vector spaces. Namely, a linear representation 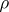of a group 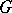is called imprimitive if there exists a decomposition of the space 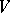of the representation 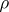into a direct sum of proper subspaces 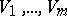with the following property: For any and any 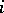, , 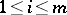there exists a 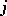, , 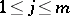such that

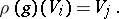The collection of subsets 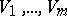is called a system of imprimitivity of the representation 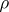. If 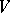does not have a decomposition of the above type, then 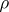is said to be a primitive representation. An imprimitive representation 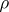is called transitive imprimitive if there exists for any pair of subspaces 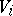and 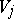of the system of imprimitivity an element 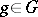such that 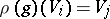. The group 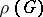of linear transformations of the space 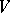and the 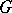-module 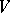defined by the representation are also called imprimitive (or primitive) if the representation 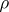is imprimitive (or primitive).

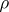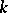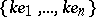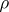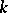Examples. A representation of the symmetric group in the -dimensional vector space over a field that rearranges the elements of a basis is transitive imprimitive, the one-dimensional subspaces form a system of imprimitivity for . Another example of a transitive imprimitive representation is the regular representation of a finite group over a field ; the collection of one-dimensional subspaces 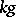, where 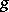runs through , forms a system of imprimitivity. More generally, any monomial representation of a finite group is imprimitive. The representation of a cyclic group of order 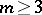by rotations of the real plane through angles that are multiples of 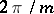is primitive.

The notion of an imprimitive representation is closely related to that of an induced representation. Namely, let 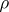be an imprimitive finite-dimensional representation of a finite group with system of imprimitivity 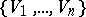. The set 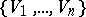is partitioned into a union of orbits with respect to the action of 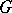determined by 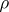. Let 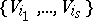be a complete set of representatives of the different orbits of this action, let

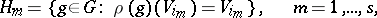let 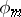be the representation of the group 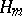in 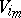defined by the restriction of 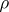to 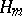, and let 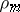be the representation of 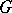induced by 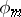. Then is equivalent to the direct sum of the representations 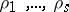. Conversely, let 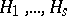be any collection of subgroups of , let 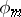be a representation of in a finite-dimensional vector space 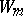, , 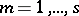and let be the representation of 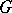induced by 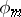. Suppose further that 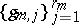is a system of representatives of left cosets of 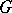with respect to 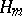. Then the direct sum of the representations is imprimitive, while 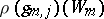, , , 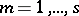is a system of imprimitivity (here 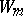is canonically identified with a subspace of ).

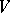References

[1]	M. Hall, "Group theory" , Macmillan (1959)
[2]	C.W. Curtis, I. Reiner, "Representation theory of finite groups and associative algebras" , Interscience (1962)

Comments

A domain of imprimitivity is also called a block.