Idempotent

idempotent element

An element of a ring, semi-group or groupoid equal to its own square: . An idempotent is said to contain an idempotent (denoted by ) if . For associative rings and semi-groups, the relation is a partial order on the set of idempotent elements, called the natural partial order on . Two idempotents and of a ring are said to be orthogonal if 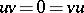. With every idempotent of a ring (and also with every system of orthogonal idempotents) there is associated the so-called Peirce decomposition of the ring. For an -ary algebraic relation , an element is said to be an idempotent if , where occurs times between the brackets.

Comments

An algebraic operation is sometimes said to be idempotent if every element of the set on which it acts is idempotent in the sense defined above. Such operations are also called affine operations; the latter name is preferable because an affine unary operation is not the same thing as an idempotent element of the semi-group of unary operations. In the theory of 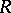-modules, the affine operations are those of the form

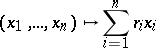with .