Irreducible module

simple module

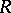A non-zero unitary module over a ring with a unit element that contains only two submodules: the null module and itself.

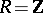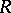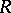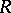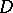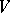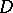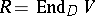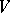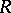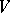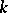Examples. 1) If is the ring of integers, then the irreducible -modules are the Abelian groups of prime order. 2) If is a skew-field, then the irreducible -modules are the one-dimensional vector spaces over . 3) If is a skew-field, is a left vector space over and is the ring of linear transformations of (or a dense subring of it), then the right -module is irreducible. 4) If is a group and is a field, then the irreducible representations of 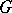over are precisely the irreducible modules over the group algebra .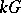

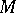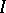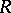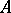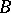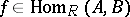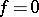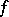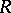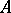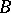A right -module is irreducible if and only if it is isomorphic to , where is a maximal right ideal in . If and are irreducible -modules and , then either or is an isomorphism (which implies that the endomorphism ring of an irreducible module is a skew-field). If is an algebra over an algebraically closed field and if and are irreducible modules over 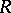, then (Schur's lemma)

The concept of an irreducible module is fundamental in the theories of rings and group representations. By means of it one defines the composition sequence and the socle of a module, the Jacobson radical of a module and of a ring, and a completely-reducible module. Irreducible modules are involved in the definition of a number of important classes of rings: classical semi-simple rings, primitive rings, and others (cf. Classical semi-simple ring; Primitive ring).

References

[1]	N. Jacobson, "Structure of rings" , Amer. Math. Soc. (1956)
[2]	C.W. Curtis, I. Reiner, "Representation theory of finite groups and associative algebras" , Interscience (1962)
[3]	J. Lambek, "Lectures on rings and modules" , Blaisdell (1966)
[4]	C. Faith, "Algebra: rings, modules, and categories" , 1–2 , Springer (1973–1976)