Free magma
From Encyclopedia of Mathematics
Let 
be a set. Define sets 
, 
, inductively as follows:
 |
 |
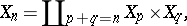where 
denotes the disjoint union (see Union of sets). Let
 |
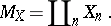There is an obvious binary relation on 
: if 
, 
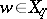, then the pair 
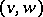goes to the element 
of 
. This is the free magma on 
. It has the obvious freeness property: if 
is any magma and 
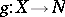is a function, then there is a unique morphism of magmas 
extending 
.
Certain special subsets of 
, called Hall sets (cf. Hall set), are important in combinatorics and the theory of Lie algebras.
The free magma over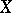 
can be identified with the set of binary complete, planar, rooted trees with leaves labelled by 
. See Binary tree.
References
| [a1] | N. Bourbaki, "Groupes et algèbres de Lie" , 2: Algèbres de Lie libres , Hermann (1972) |
| [a2] | C. Reutenauer, "Free Lie algebras" , Oxford Univ. Press (1993) |
| [a3] | J.-P. Serre, "Lie algebras and Lie groups" , Benjamin (1965) |
How to Cite This Entry:
Free magma. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Free_magma&oldid=12862
Free magma. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Free_magma&oldid=12862
This article was adapted from an original article by M. Hazewinkel (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article