Cartan subalgebra

of a finite-dimensional Lie algebra 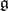over a field

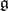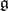A nilpotent subalgebra of which is equal to its normalizer in . For example, if is the Lie algebra of all complex square matrices of a fixed order, then the subalgebra of all diagonal matrices is a Cartan subalgebra in 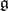. A Cartan subalgebra can also be defined as a nilpotent subalgebra 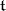in which is equal to its Fitting null-component (cf. Weight of a representation of a Lie algebra)

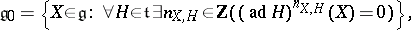where 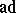denotes the adjoint representation (cf. Lie algebra) of .

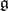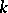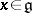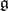Suppose further that is of characteristic zero. Then for any regular element , the set of all elements of which are annihilated by powers of 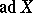is a Cartan subalgebra of 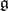, and every Cartan subalgebra of 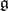has the form 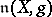for some suitable regular element 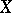. Each regular element belongs to one and only one Cartan subalgebra. The dimension of all the Cartan subalgebras of 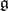are the same and are equal to the rank of . The image of a Cartan subalgebra under a surjective homomorphism of Lie algebras is a Cartan subalgebra. If 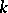is algebraically closed, then all Cartan subalgebras of 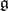are conjugate; more precisely, they can be transformed into another by operators of the algebraic group 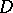of automorphisms of 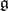whose Lie algebra is the commutator subalgebra o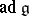f . If 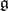is solvable, then the above assertion holds without the hypothesis that 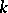be algebraically closed.

Let be either a connected linear algebraic group over an algebraically closed field 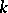of characteristic zero, or a connected Lie group, and let 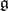be its Lie algebra. Then a subalgebra 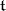of 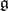is a Cartan subalgebra if and only if it is the Lie algebra of a Cartan subgroup of .

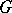Let 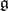be a subalgebra of the Lie algebra 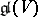of all endomorphisms of a finite-dimensional vector space 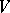over 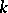, and let 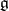be the smallest algebraic Lie algebra in containing 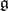(cf. Lie algebra, algebraic). If 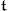is a Cartan subalgebra of 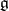, then 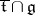is a Cartan subalgebra of 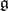, and if 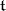is a Cartan subalgebra of 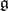and 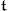is the smallest algebraic subalgebra of 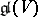containing 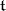, then 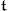is a Cartan subalgebra of 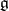and .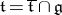

Let 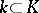be a field extension. A subalgebra of 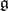is a Cartan subalgebra if and only if 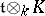is a Cartan subalgebra of .

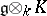Cartan subalgebras play an especially important role when 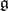is a semi-simple Lie algebra (this was used by E. Cartan [1]). In this case, every Cartan subalgebra 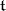of 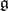is Abelian and consists of semi-simple elements (see Jordan decomposition), and the restriction of the Killing form to 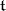is non-singular.

References

[1]	E. Cartan, "Sur la structure des groupes de transformations finis et continus" , Paris (1894)
[2]	N. Jacobson, "Lie algebras" , Interscience (1962) ((also: Dover, reprint, 1979))
[3]	C. Chevalley, "Theory of Lie groups" , 1 , Princeton Univ. Press (1946)
[4]	, Theórie des algèbres de Lie. Topologie des groupes de Lie , Sem. S. Lie , Ie année 1954–1955 , Ecole Norm. Sup. (1955)

Comments

An element 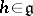is called regular if the dimension of the Fitting null-component of the endomorphism 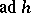of 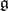is minimal. "Almost-all" elements of 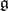are regular in the sense that the condition of being regular defines a Zariski-open subset. The result that the Fitting null-component of 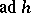for regular is a Cartan subalgebra holds for finite-dimensional Lie algebras over any infinite field [a4], p. 59.

References

[a1]	N. Bourbaki, "Elements of mathematics. Lie groups and Lie algebras" , Addison-Wesley (1975) (Translated from French)
[a2]	J.E. Humphreys, "Introduction to Lie algebras and representation theory" , Springer (1972) pp. §5.4
[a3]	J.-P. Serre, "Lie algebras and Lie groups" , Benjamin (1965) (Translated from French)
[a4]	N. Jacobson, "Lie algebras" , Dover, reprint (1979) ((also: Dover, reprint, 1979))