Free set
in a vector space 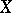 over a field
over a field 
A linearly independent system of vectors from 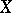 , that is, a set of elements
, that is, a set of elements 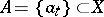 ,
, 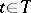 , such that the relation
, such that the relation 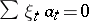 , where
, where  for all but a finite number of indices
for all but a finite number of indices 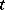 , implies that
, implies that 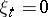 for all
for all 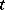 . A non-free set is also called dependent.
. A non-free set is also called dependent.
A free set in a topological vector space 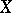 over a field
over a field 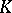 (a topologically-free set) is a set
(a topologically-free set) is a set 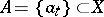 such that for any
such that for any  the closed subspace generated by the points
the closed subspace generated by the points 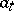 ,
, 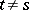 , does not contain
, does not contain 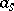 . A topologically-free set is a free set in the vector space; the converse is not true. For example, in the normed space
. A topologically-free set is a free set in the vector space; the converse is not true. For example, in the normed space 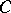 of continuous functions on
of continuous functions on 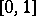 , the functions
, the functions 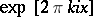 ,
,  , form a topologically-free set, in contrast to the functions
, form a topologically-free set, in contrast to the functions 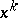 (since, e.g.,
(since, e.g.,  is contained in the closed subspace generated by
is contained in the closed subspace generated by 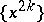 ).
).
The set of all (topologically-) free sets in 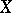 is, in general, not inductive under inclusion; in addition, it does not necessarily contain a maximal topologically-free set. For example, let
is, in general, not inductive under inclusion; in addition, it does not necessarily contain a maximal topologically-free set. For example, let 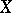 be the space over
be the space over 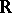 formed by the continuous functions and endowed with the following Hausdorff topology: a fundamental system of neighbourhoods of zero in
formed by the continuous functions and endowed with the following Hausdorff topology: a fundamental system of neighbourhoods of zero in  consists of the balanced absorbing sets
consists of the balanced absorbing sets 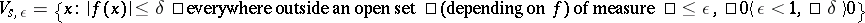 . Then every continuous linear functional vanishes, and
. Then every continuous linear functional vanishes, and 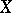 does not contain a maximal free set.
does not contain a maximal free set.
For 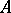 to be a (topologically-) free set in the weak topology
to be a (topologically-) free set in the weak topology 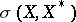 in
in 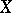 it is necessary and sufficient that for each
it is necessary and sufficient that for each  there is a
there is a 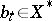 such that
such that  , and
, and 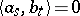 for all
for all 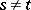 . For a locally convex space a free set in the weak topology is a free set in the original topology.
. For a locally convex space a free set in the weak topology is a free set in the original topology.
Free set. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Free_set&oldid=12183