Profinite group

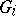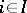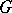A topological group that is the projective limit of an inverse system of finite discrete groups , (where is a pre-ordered directed set). The profinite group is denoted by 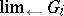. As a subspace of the direct product , endowed with the compact topology (a neighbourhood base of the identity is given by the system of kernels of the projections 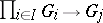), it is closed and hence compact.

Examples.

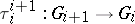1) Let be the set of integers larger than zero with the natural order relation, and let . Suppose that is the natural epimorphism, and put

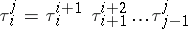for all 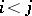. Then 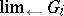is the (additive) group of the ring of 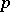-adic integers.

2) Every compact analytic group over a 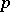-adic number field (e.g. 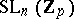) is profinite as a topological group.

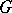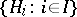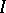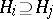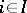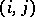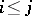3) Let be an abstract group and let be the family of its normal subgroups of finite index. On one introduces the relation , putting if . This relation turns into a pre-ordered directed set. Associate to the group , and to each pair , , the natural homomorphism 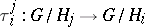. One obtains the profinite group 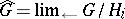, called the profinite group completion of 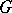. It is the separable completion of 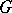(cf. Separable completion of a ring) for the topology defined by the subgroups of finite index. The kernel of the natural homomorphism 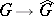is the intersection of all subgroups of finite index. In this construction one can consider, instead of the family of all normal subgroups of finite index, only those whose index is a fixed power of a prime number 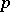. The corresponding group is denoted by 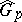, and is a pro-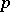-group.

4) Profinite groups naturally arise in Galois theory of (not necessarily finite) algebraic extensions of fields in the following way. Let 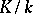be a Galois extension and suppose that 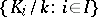is the family of all finite Galois extensions of 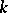lying in 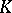. Then , and one can introduce on 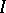the relation 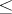by putting 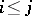if 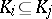. The set 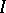then becomes pre-ordered. Let 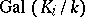be the Galois group of 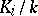. To every pair 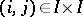, , 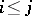one naturally associates the homomorphism

The corresponding profinite group 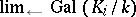is isomorphic to 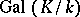, thus 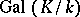can be considered as a profinite group. The system forms in a neighbourhood base of the identity (cf. Galois topological group). This construction has a generalization in algebraic geometry in the definition of the fundamental group of a scheme.

A profinite group can be characterized as a compact totally-disconnected group (cf. Compact group), as well as a compact group that has a system of open normal subgroups forming a neighbourhood base of the identity. The cohomology theory of profinite groups (cf. Cohomology of groups; Galois cohomology) plays an important role in modern Galois theory.

References

[1]	J.-P. Serre, "Cohomologie Galoisienne" , Springer (1964)
[2]	H. Koch, "Galoissche Theorie der 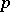-Erweiterungen" , Deutsch. Verlag Wissenschaft. (1970)
[3]	J.W.S. Cassels (ed.) A. Fröhlich (ed.) , Algebraic number theory , Acad. Press (1986)