Evolute

of a plane curve

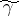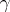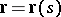The set of the centres of curvature of the given curve . If (where is the arc length parameter of 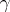) is the equation of 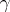, then the equation of its evolute has the form

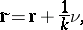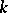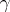where is the curvature and the unit normal to . The figures shows the construction of the evolute in three typical cases:

a) if along the entire curve 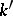has a fixed sign and 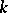does not vanish;

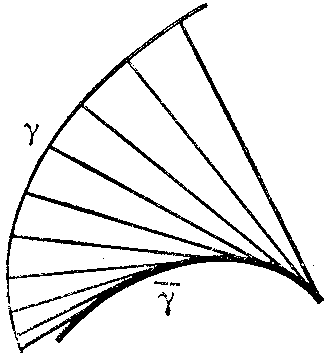
Figure: e036670a

Figure: e036670b

b) if along the entire curve has a fixed sign and 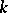vanishes for 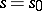; and

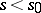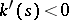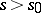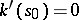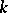c) if for ; for ; , and does not vanish (the point of the evolute corresponding to is a cusp).

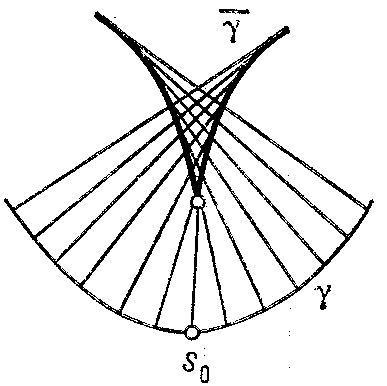
Figure: e036670c

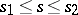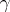The length of the arc of the evolute corresponding to the segment of is

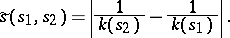The evolute is the envelope of the normals to 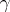. The curve 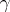is called the evolvent of its evolute (cf. Evolvent of a plane curve).

Comments

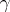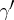The evolvent is also called the involute; thus, if is the evolute of , then is the involute of , cf. Evolvent of a plane curve.

References

[a1]	H.-R. Müller, "Kinematik" , de Gruyter (1963)
[a2]	M. Berger, B. Gostiaux, "Differential geometry: manifolds, curves, and surfaces" , Springer (1988) pp. 305 (Translated from French)
[a3]	J.L. Coolidge, "Algebraic plane curves" , Dover, reprint (1959) pp. 195
[a4]	M. Berger, "Geometry" , I , Springer (1987) pp. 253–254
[a5]	H.W. Guggenheimer, "Differential geometry" , McGraw-Hill (1963) pp. 25; 60