Paraboloidal coordinates
From Encyclopedia of Mathematics
Numbers 
, 
and 
related to the rectangular Cartesian coordinates 
, 
and 
by the formulas
 |
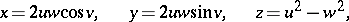where 
, 
, 
. The coordinate surfaces are two systems of paraboloids of revolution with oppositely-directed axes (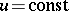
and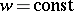 
) and half-planes (
). The system of paraboloidal coordinates is orthogonal.
The Lamé coefficients (or scale factors) are
 |
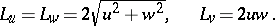The element of surface area is
 |
 |
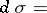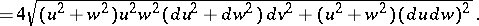The volume element is
 |
The fundamental operations of vector analysis are
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
 |
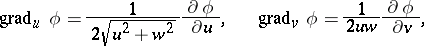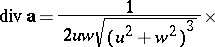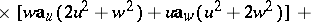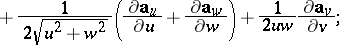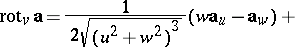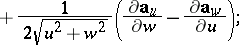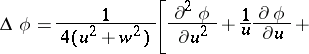Comments
These coordinates are also called rotation parabolic coordinates.
References
| [a1] | R. Sauer (ed.) I. Szabó (ed.) , Mathematische Hilfsmittel des Ingenieurs , 1 , Springer (1967) pp. 96 |
How to Cite This Entry:
Paraboloidal coordinates. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Paraboloidal_coordinates&oldid=11852
Paraboloidal coordinates. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Paraboloidal_coordinates&oldid=11852
This article was adapted from an original article by D.D. Sokolov (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article