Grothendieck functor
An imbedding functor (cf. Imbedding of categories) from a category 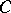 into the category
into the category  of contravariant functors defined on
of contravariant functors defined on 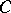 and taking values in the category of sets (Ens). Let
and taking values in the category of sets (Ens). Let 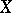 be an object in a category
be an object in a category 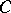 ; the mapping
; the mapping 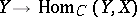 defines a contravariant functor
defines a contravariant functor 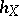 from
from 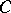 into the category of sets. For any object
into the category of sets. For any object  of
of 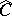 there exists a natural bijection
there exists a natural bijection  (Yoneda's lemma). Hence, in particular
(Yoneda's lemma). Hence, in particular
 |
Accordingly, the mapping  defines a full imbedding
defines a full imbedding 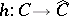 , which is known as the Grothendieck functor. Using the Grothendieck functor it is possible to define algebraic structures on objects of a category (cf. Group object; Group scheme).
, which is known as the Grothendieck functor. Using the Grothendieck functor it is possible to define algebraic structures on objects of a category (cf. Group object; Group scheme).
References
| [1] | I. Bucur, A. Deleanu, "Introduction to the theory of categories and functors" , Wiley (1968) |
| [2] | A. Grothendieck, "Technique de descente et théorèmes d'existence en géométrie algébrique, II" Sem. Bourbaki , Exp. 195 (1960) |
Comments
In the English literature, the Grothendieck functor is commonly called the Yoneda embedding or the Yoneda–Grothendieck embedding.
References
| [a1] | S. MacLane, "Categories for the working mathematician" , Springer (1971) pp. Chapt. IV, Sect. 6; Chapt. VII, Sect. 7 |
| [a2] | N. Yoneda, "On the homology theory of modules" J. Fac. Sci. Tokyo. Sec. I , 7 (1954) pp. 193–227 |
Grothendieck functor. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Grothendieck_functor&oldid=11791