Anti-de Sitter space
complete maximal space-like hypersurfaces in an
Let  be an
be an 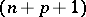 -dimensional Minkowski space of index
-dimensional Minkowski space of index 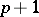 , i.e.,
, i.e.,  and is equipped with the Lorentz metric
and is equipped with the Lorentz metric  . For
. For 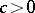 , let
, let
 |
 |
Thus, 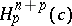 is an
is an  -dimensional indefinite Riemannian manifold of index
-dimensional indefinite Riemannian manifold of index 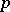 and of constant curvature
and of constant curvature 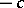 . It is called an
. It is called an  -dimensional anti-de Sitter space of constant curvature
-dimensional anti-de Sitter space of constant curvature 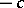 and of index
and of index 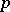 . A hypersurface
. A hypersurface  of
of 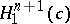 is said to be space-like if the metric on
is said to be space-like if the metric on  induced by that of ambient space
induced by that of ambient space  is positive definite. The mean curvature
is positive definite. The mean curvature 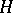 of
of  is defined as in the case of Riemannian manifolds. By definition,
is defined as in the case of Riemannian manifolds. By definition,  is a maximal hypersurface if the mean curvature
is a maximal hypersurface if the mean curvature 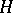 of
of  is identically zero. S. Ishihara proved that a complete maximal space-like hypersurface
is identically zero. S. Ishihara proved that a complete maximal space-like hypersurface  in
in 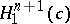 satisfies
satisfies  , and
, and 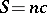 if and only if
if and only if  is isometric to the hyperbolic cylinder
is isometric to the hyperbolic cylinder  , where
, where 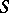 is the squared norm of the second fundamental form of
is the squared norm of the second fundamental form of  and
and  ,
, 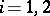 , is a
, is a 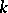 -dimensional hyperbolic space of constant curvature
-dimensional hyperbolic space of constant curvature 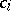 . The rigidity of the hyperbolic cylinder
. The rigidity of the hyperbolic cylinder  in
in  was proved by U.-H. Ki, H.S. Kim and H. Nakagawa [a3]: for a given integer
was proved by U.-H. Ki, H.S. Kim and H. Nakagawa [a3]: for a given integer  and constant
and constant 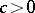 , there exists a constant
, there exists a constant 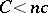 , depending on
, depending on  and
and  , such that the hyperbolic cylinder
, such that the hyperbolic cylinder  is the only complete maximal space-like hypersurface in
is the only complete maximal space-like hypersurface in 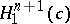 of constant scalar curvature and such that
of constant scalar curvature and such that  . In particular, for
. In particular, for 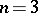 , Q.M. Cheng [a1] has characterized the complete maximal space-like hypersurfaces in
, Q.M. Cheng [a1] has characterized the complete maximal space-like hypersurfaces in  under the condition of constant Gauss–Kronecker curvature (cf. Gaussian curvature): Let
under the condition of constant Gauss–Kronecker curvature (cf. Gaussian curvature): Let  be a
be a  -dimensional complete maximal space-like hypersurface of
-dimensional complete maximal space-like hypersurface of  . Now:
. Now:
1) if the Gauss–Kronecker curvature of  is a non-zero constant, then
is a non-zero constant, then  is the hyperbolic cylinder
is the hyperbolic cylinder  ;
;
2) if the scalar curvature 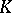 is constant and
is constant and  , then
, then  is the hyperbolic cylinder
is the hyperbolic cylinder 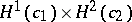 . There are no complete maximal space-like hypersurfaces in
. There are no complete maximal space-like hypersurfaces in  with constant scalar curvature and
with constant scalar curvature and 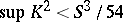 .
.
On the other hand, complete space-like submanifolds in anti-de Sitter spaces with parallel mean curvature have been investigated by many authors.
Cf. also De Sitter space.
References
| [a1] | Q.M. Cheng, "Complete maximal space-like hypersurfaces of 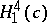 " Manuscr. Math. , 82 (1994) pp. 149–160 " Manuscr. Math. , 82 (1994) pp. 149–160 |
| [a2] | T. Ishikawa, "Maximal space-like submanifolds of a pseudo–Riemannian space of constant curvature" Michigan Math. J. , 35 (1988) pp. 345–352 |
| [a3] | U-H. Ki, H.S. Kim, H. Nakagawa, "Complete maximal space-like hypersurfaces of an anti-de Sitter space" Kyungpook Math. J. , 31 (1991) pp. 131–141 |
Anti-de Sitter space. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Anti-de_Sitter_space&oldid=11698