Bicategory(2)
A category  in which subcategories of epimorphisms
in which subcategories of epimorphisms 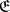 and of monomorphisms
and of monomorphisms 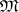 have been distinguished such that the following conditions are met:
have been distinguished such that the following conditions are met:
1) all morphisms 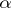 in
in  are decomposable into a product
are decomposable into a product 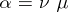 , where
, where  ,
,  ;
;
2) if  , where
, where 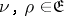 ,
, 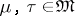 , then there exists an isomorphism
, then there exists an isomorphism 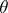 such that
such that 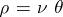 , and
, and 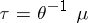 ;
;
3) 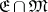 coincides with the class of isomorphisms in the category
coincides with the class of isomorphisms in the category 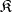 .
.
The epimorphisms in  (the monomorphisms in
(the monomorphisms in 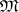 ) are called the permissible epimorphisms (monomorphisms) of the bicategory.
) are called the permissible epimorphisms (monomorphisms) of the bicategory.
The concept of a bicategory axiomatizes the possibility of a decomposition of an arbitrary mapping into a product of a surjective and an injective mapping. The category of sets, the category of sets with a marked point and the category of groups are bicategories with a unique bicategorical structure. In the category of all topological spaces and in the category of all associative rings there are proper classes of different bicategorical structures.
References
| [1] | M.Sh. Tsalenko, E.G. Shul'geifer, "Fundamentals of category theory" , Moscow (1974) (In Russian) |
Comments
In the literature there has been much confusion about the terms bicategory and  -category. Usually, bicategory is understood to mean "generalized 2-category" , and a bicategory as defined above is called, e.g., "bicategory in the sense of Isbell" .
-category. Usually, bicategory is understood to mean "generalized 2-category" , and a bicategory as defined above is called, e.g., "bicategory in the sense of Isbell" .
In this Encyclopaedia the term bicategory is always used as defined above.
Bicategory(2). Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Bicategory(2)&oldid=11447