Star body
with respect to a point 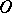 , star-like body
, star-like body
An open set 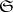 in
in  -dimensional Euclidean space
-dimensional Euclidean space 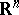 which has the ray property (relative to
which has the ray property (relative to  ): If
): If  , where
, where 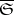 is the closure of
is the closure of 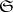 , then the entire segment
, then the entire segment 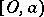 (where
(where  ,
, 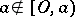 ) lies in
) lies in 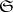 . A star body
. A star body 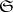 with centre
with centre 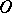 may be characterized as follows:
may be characterized as follows: 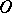 is an interior point of
is an interior point of 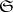 ; every ray emanating from
; every ray emanating from 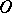 lies either entirely in
lies either entirely in 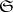 or contains a point
or contains a point  such that the ray segment
such that the ray segment 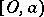 lies in
lies in 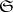 , but the ray segment
, but the ray segment 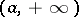 lies outside
lies outside 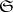 . This definition is equivalent to the first one, up to points on the boundary of
. This definition is equivalent to the first one, up to points on the boundary of 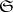 . A star body is a particular case of a star set with respect to
. A star body is a particular case of a star set with respect to 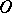 , a set with the generalized ray property relative to
, a set with the generalized ray property relative to 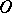 : If
: If  , then the entire segment
, then the entire segment 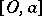 lies in
lies in 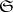 . A particular case of a star body is a convex body.
. A particular case of a star body is a convex body.
With every star body 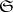 with respect to the origin
with respect to the origin 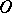 one can associate, in one-to-one fashion, a ray function
one can associate, in one-to-one fashion, a ray function 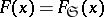 such that
such that 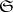 is the set of points
is the set of points  with
with 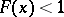 .
.
The correspondence is defined by the formula
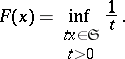 |
With this notation a star body 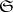 is bounded if and only if
is bounded if and only if  is a positive ray function; it is convex if and only if
is a positive ray function; it is convex if and only if 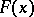 is a convex ray function.
is a convex ray function.
References
| [1] | J.W.S. Cassels, "An introduction to the geometry of numbers" , Springer (1972) |
Comments
Star bodies play an important role in the geometry of numbers, e.g. the Minkowski–Hlawka theorem.
A set  in
in  is centrally symmetric if
is centrally symmetric if  implies
implies 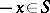 .
.
The Minkowski–Hlawka theorem says that 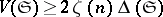 for a centrally-symmetric star body
for a centrally-symmetric star body 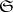 . Here,
. Here, 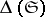 is the critical determinant of
is the critical determinant of 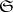 (cf. Geometry of numbers),
(cf. Geometry of numbers), 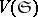 is the volume of
is the volume of 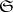 and
and  . This is an inequality in the opposite direction of the Minkowski convex body theorem (cf. Minkowski theorem).
. This is an inequality in the opposite direction of the Minkowski convex body theorem (cf. Minkowski theorem).
References
| [a1] | P.M. Gruber, C.G. Lekkerkerker, "Geometry of numbers" , North-Holland (1987) pp. Sect. (iv) (Updated reprint) |
| [a2] | P. Erdös, P.M. Gruber, J. Hammer, "Lattice points" , Longman (1989) |
Star body. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Star_body&oldid=11275